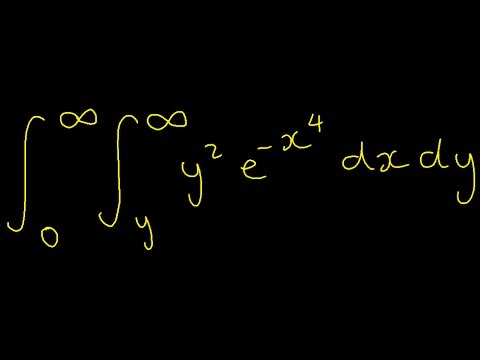The integral of x^e^x^2 is given by, ∫xe x 2 dx = (1/2) e x 2 + C. This value can be determined by substituting the limits and 0 and 1 into the formula ∫xe x dx = e x(x - 1) + C and find its value.
The definite integral of xe^x from 0 to 1 is equal to 1. What is the Definite Integral of xe^x From 0 to 1? We can use the formula of integration by parts given by, ∫f(x) g(x) dx = f(x) ∫g(x) dx - ∫ dx OR ∫udv = uv - ∫vdu. We can find the integral of xe x using one of the most commonly used and important methods of integration known as the integration by parts. We can calculate this integral by using the ILATE sequence of function in integration by parts method.
The formula for the integration of xe x is given by, ∫xe x dx = xe x - e x + C (OR) ∫xe x dx = e x(x - 1) + C, where C is the integration constant. We can evaluate this integral using the integration by parts formula. The integral of xe x gives the area under the curve of the function f(x) = xe x. The integral of xe x is equal to xe x - e x + C, where C is the constant of integration. FAQs on Integral of xe x What is Integral of xe x in Calculus?